I've done a number of activities over the years for YH"A, some of which I will share in the future. This year's activity is quite beta. There are challenges inherent in doing it properly, which I'll explain later. I generated this set of instructions that utilize the skills my 6th graders have already mastered.
The skills are:
a) Plot points on a coordinate plane.
b) Identify points that are reflections over the x-axis and the y-axis.
c) *Challenge only: Calculate the area of the triangles that form the Magen David on the flag.
If you just want the worksheet, it is here in PDF and Word.
Screenshots appear at the bottom of the post.
When they are done, their picture should look like an Israeli flag.
(Image from the Wikimedia Commons.)
I started by buying some of these big coordinate grid pads. The kind that are sticky on the back are much more expensive. These are cheaper, although I recognize coordinate grid paper might be outside of some school's budgets. (You can use this activity on a normal piece of graph paper, too.)
I started my making giant axes for the students. For my weakest students I'll label the axis with its numbers, but for the other students, I just made the x-axis and y-axis.
I went through several drafts of the coordinates. The triangles must be equilateral, which means that we use a 30-60-90 triangle to find the side lengths. (Each main triangle on the Magen David is made up of two triangles like the one that appears below.)
(Image from the Wikimedia Commons.)
In a 30-60-90 triangle, if the height is rational, the side length is irrational and vice versa. This means that we have to round if we want the height ( a*root 3) and the side length (2a) to be integers. I approximated by making the side length 14. That makes a=7, which works out to a height of ~12.12.
I stumbled upon this excellent choice - excellent because the height of 12 is divisible by 3, and each triangle is 3/4 of the height of the total Magen David. (As always, I owe this particular insight to my estimable colleague John Watkins-Chow.)
The way I generated my triangle was to choose a top point, use a protractor to center a 60 degree angle around that point, and then create a side length that was close to a lattice point.
Then I moved up or down to center appropriately. I redid it if I thought the star was too large for the page or if it was not centered. I eventually arrived at the points I chose here.
If I was doing this with 8th graders, I would change #3-6 to graphing linear inequalities.
I'll discover on Tuesday how this works, but I encourage anyone who is interested to send me a shot of their graphs that they create.
Chag Ha'atzmaut Sameach!
Edits: The first version of this post neglected to mention John Watkins-Chow's assistance.









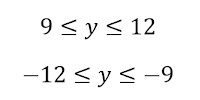
No comments:
Post a Comment